| Issue |
ESAIM: COCV
Volume 8, 2002
A tribute to JL Lions
|
|
|---|---|---|
| Page(s) | 69 - 103 | |
| DOI | https://doi.org/10.1051/cocv:2002018 | |
| Published online | 15 August 2002 | |
Some regularity results for minimal crystals
1
Scuola Normale Superiore,
Piazza dei Cavalieri 7, 56126 Pisa, Italy; ambrosio@emath.ethz.ch.
2
Dipartimento di Matematica, Università di Pisa,
via F. Buonarroti 2, 56126 Pisa, Italy.
Received:
4
October
2001
We introduce an intrinsic notion of perimeter for subsets of
a general Minkowski space (i.e. a finite dimensional Banach space in which the
norm is not required to be even).
We prove that this notion of perimeter is equivalent to
the usual definition of surface energy for crystals and
we study the regularity properties of
the minimizers and the quasi-minimizers of perimeter.
In the two-dimensional case we obtain optimal regularity results:
apart from a singular set (which is 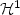 -negligible and is empty when
the unit ball is neither
a triangle nor a quadrilateral), we find that quasi-minimizers can be locally
parameterized by means of a bi-lipschitz curve, while sets
with prescribed bounded curvature are, locally, lipschitz graphs.
-negligible and is empty when
the unit ball is neither
a triangle nor a quadrilateral), we find that quasi-minimizers can be locally
parameterized by means of a bi-lipschitz curve, while sets
with prescribed bounded curvature are, locally, lipschitz graphs.
Mathematics Subject Classification: 49J45 / 49Q20
Key words: Quasi-minimal sets / Wulff shape / crystalline norm.
© EDP Sciences, SMAI, 2002
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


