| Issue |
ESAIM: COCV
Volume 11, Number 4, October 2005
|
|
|---|---|---|
| Page(s) | 522 - 541 | |
| DOI | https://doi.org/10.1051/cocv:2005021 | |
| Published online | 15 September 2005 | |
On ergodic problem for Hamilton-Jacobi-Isaacs equations
SISSA/ISAS via Beirut, 2-4 - 34013 Trieste, Italy; bettiol@ma.sissa.it
Received:
20
January
2004
Revised:
3
November
2004
We study the asymptotic behavior of 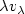 as
as
 , where
, where  is the viscosity solution of the following Hamilton-Jacobi-Isaacs
equation (infinite horizon case)
is the viscosity solution of the following Hamilton-Jacobi-Isaacs
equation (infinite horizon case)
![\[ \lambda v_\lambda + H(x,Dv_\lambda)=0, \]](/articles/cocv/abs/2005/04/cocv0406/cocv0406_tex_eq4.png) with
with
![\[ H(x,p):=\min_{b\in B}\max_{a \in A} \{-f(x,a,b)\cdot p -l(x,a,b)\}. \]](/articles/cocv/abs/2005/04/cocv0406/cocv0406_tex_eq5.png) We discuss the cases in which the state of the system is required to stay in an
n-dimensional torus, called periodic boundary conditions,
or in the closure
of a bounded connected domain
We discuss the cases in which the state of the system is required to stay in an
n-dimensional torus, called periodic boundary conditions,
or in the closure
of a bounded connected domain 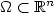 with sufficiently smooth boundary.
As far as the latter is concerned, we treat
both
the case of the Neumann boundary conditions
(reflection on the boundary) and
the case of state constraints boundary conditions.
Under the uniform approximate controllability
assumption of one player, we extend
the uniform convergence result of the value function to a constant as
with sufficiently smooth boundary.
As far as the latter is concerned, we treat
both
the case of the Neumann boundary conditions
(reflection on the boundary) and
the case of state constraints boundary conditions.
Under the uniform approximate controllability
assumption of one player, we extend
the uniform convergence result of the value function to a constant as
 to differential games.
As far as state constraints boundary conditions are concerned,
we give an example where the value function is Hölder continuous.
to differential games.
As far as state constraints boundary conditions are concerned,
we give an example where the value function is Hölder continuous.
Mathematics Subject Classification: 35B40 / 49L25 / 49N70
Key words: Hamilton-Jacobi-Isaacs equations / viscosity solutions / asymptotic behavior / differential games / boundary conditions / ergodicity.
© EDP Sciences, SMAI, 2005
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


