| Issue |
ESAIM: COCV
Volume 7, 2002
|
|
|---|---|---|
| Page(s) | 335 - 377 | |
| DOI | https://doi.org/10.1051/cocv:2002015 | |
| Published online | 15 September 2002 | |
Stabilization of the wave equation by on-off and positive-negative feedbacks
M.I.P. Université Paul Sabatier Toulouse III,
118 route de Narbonne, 31062 Toulouse Cedex 4, France; martinez@mip.ups-tlse.fr.,vancoste@mip.ups-tlse.fr.
Received:
25
June
2001
Revised:
27
November
2001
Motivated by several works on the stabilization of the oscillator by
on-off feedbacks, we study the related problem for the one-dimensional wave equation, damped
by an on-off feedback  .
We obtain results that are radically different from those known in the case
of the oscillator. We consider periodic functions a: typically
a is equal to 1 on (0,T),
equal to 0 on (T, qT) and is qT-periodic.
We study the boundary case and next the locally distributed case,
and we give optimal results of stability. In both cases,
we prove that there are explicit exceptional values of T
for which the energy of some solutions remains constant with time. If
T is different from those exceptional values, the energy of all solutions
decays exponentially to zero. This number of exceptional values is
countable in the boundary case and
finite in the distributed case.
When the feedback is acting on the boundary,
we also study the case
of postive-negative feedbacks:
.
We obtain results that are radically different from those known in the case
of the oscillator. We consider periodic functions a: typically
a is equal to 1 on (0,T),
equal to 0 on (T, qT) and is qT-periodic.
We study the boundary case and next the locally distributed case,
and we give optimal results of stability. In both cases,
we prove that there are explicit exceptional values of T
for which the energy of some solutions remains constant with time. If
T is different from those exceptional values, the energy of all solutions
decays exponentially to zero. This number of exceptional values is
countable in the boundary case and
finite in the distributed case.
When the feedback is acting on the boundary,
we also study the case
of postive-negative feedbacks:  on (0,T),
and
on (0,T),
and 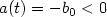 on (T,qT), and we give the necessary and
sufficient condition
under which the energy (that is no more nonincreasing with time) goes
to zero or goes to infinity.
The proofs of these results
are based on congruence properties and on a theorem of Weyl in the
boundary case, and on
new observability inequalities for the undamped wave equation,
weakening the usual “optimal time condition” in the locally distributed case.
These new inequalities provide also new exact controllability results.
on (T,qT), and we give the necessary and
sufficient condition
under which the energy (that is no more nonincreasing with time) goes
to zero or goes to infinity.
The proofs of these results
are based on congruence properties and on a theorem of Weyl in the
boundary case, and on
new observability inequalities for the undamped wave equation,
weakening the usual “optimal time condition” in the locally distributed case.
These new inequalities provide also new exact controllability results.
Mathematics Subject Classification: 35L05 / 35B35 / 35B40 / 11A07
Key words: Damped wave equation / asymptotic behavior / on-off feedback / congruences / observability inequalities.
© EDP Sciences, SMAI, 2002
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


