| Issue |
ESAIM: COCV
Volume 13, Number 4, October-December 2007
|
|
|---|---|---|
| Page(s) | 717 - 734 | |
| DOI | https://doi.org/10.1051/cocv:2007032 | |
| Published online | 20 July 2007 | |
A relaxation result for energies defined on pairs set-function and applications
1
Dip. di Matematica,
Università di Roma “Tor Vergata”,
via della Ricerca Scientifica,
00133 Roma,
Italy.
2
CMAP, École Polytechnique, CNRS,
91128 Palaiseau, France; antonin.chambolle@polytechnique.fr
3
DAP, Università di Sassari, Palazzo Pou Salit,
07041 Alghero,
Italy.
Received:
12
December
2005
Revised:
17
July
2006
We consider, in an open subset Ω of 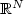 ,
energies depending on the perimeter of a subset
,
energies depending on the perimeter of a subset 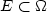 (or some equivalent surface integral) and on a function u which is
defined only on
(or some equivalent surface integral) and on a function u which is
defined only on  . We compute the lower semicontinuous envelope
of such energies. This relaxation has to take into
account the fact that in the limit, the “holes” E may
collapse into a discontinuity of u, whose surface will be counted
twice in the relaxed energy. We discuss some situations where such
energies appear, and give, as an application, a new proof
of convergence for an extension
of Ambrosio-Tortorelli's approximation to the Mumford-Shah functional.
. We compute the lower semicontinuous envelope
of such energies. This relaxation has to take into
account the fact that in the limit, the “holes” E may
collapse into a discontinuity of u, whose surface will be counted
twice in the relaxed energy. We discuss some situations where such
energies appear, and give, as an application, a new proof
of convergence for an extension
of Ambrosio-Tortorelli's approximation to the Mumford-Shah functional.
Mathematics Subject Classification: 49J45 / 49Q20 / 49Q10
Key words: Relaxation / free discontinuity problems / Γ-convergence
© EDP Sciences, SMAI, 2007
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


