| Issue |
ESAIM: COCV
Volume 16, Number 3, July-September 2010
|
|
|---|---|---|
| Page(s) | 744 - 763 | |
| DOI | https://doi.org/10.1051/cocv/2009024 | |
| Published online | 31 July 2009 | |
Hamilton-Jacobi-Bellman equations for the optimal control of a state equation with memory
Université Paris Dauphine, CEREMADE, Pl. de Lattre de Tassigny, 75775 Paris Cedex 16, France. carlier@ceremade.dauphine.fr ; tahraoui@ceremade.dauphine.fr
Received:
21
March
2008
Revised:
26
March
2009
This article is devoted to the optimal control of state equations with memory of the form:
with initial conditions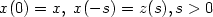 
.
Denoting by 
the solution of the previous Cauchy problem and:
where V is a class of admissible controls, we prove that v is the only viscosity solution of an Hamilton-Jacobi-Bellman equation of the form:
in the sense of the theory of viscosity solutions in infinite-dimensions of Crandall and Lions.
Mathematics Subject Classification: 49L20 / 49L25
Key words: Dynamic programming / state equations with memory / viscosity solutions / Hamilton-Jacobi-Bellman equations in infinite dimensions
© EDP Sciences, SMAI, 2009
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.