| Issue |
ESAIM: COCV
Volume 16, Number 4, October-December 2010
|
|
|---|---|---|
| Page(s) | 974 - 1001 | |
| DOI | https://doi.org/10.1051/cocv/2009033 | |
| Published online | 11 August 2009 | |
Equivalent formulation and numerical analysis of a fire confinement problem
Department of Mathematics, Penn State University
University Park, Pa. 16802, USA. bressan@math.psu.edu; wang_t@math.psu.edu
Received:
12
December
2008
Revised:
26
April
2009
We consider a class of variational
problems for differential inclusions, related to the
control of wild fires. The area burned by the fire at time t > 0
is modelled as the reachable set for
a differential inclusion  ∈ F(x), starting from
an initial set R0. To block the fire, a barrier can be constructed
progressively in time. For each t > 0, the portion of the wall constructed
within time t is described by a rectifiable set
γ(t) ⊂
∈ F(x), starting from
an initial set R0. To block the fire, a barrier can be constructed
progressively in time. For each t > 0, the portion of the wall constructed
within time t is described by a rectifiable set
γ(t) ⊂  . In this paper
we show that the search
for blocking strategies and for optimal strategies can be reduced to
a problem involving one single admissible rectifiable set Γ ⊂
. In this paper
we show that the search
for blocking strategies and for optimal strategies can be reduced to
a problem involving one single admissible rectifiable set Γ ⊂  ,
rather than the multifunction t
,
rather than the multifunction t 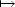 γ(t) ⊂
γ(t) ⊂  .
Relying on this result, we then develop
a numerical algorithm for the computation of
optimal strategies, minimizing the total area burned by the fire.
.
Relying on this result, we then develop
a numerical algorithm for the computation of
optimal strategies, minimizing the total area burned by the fire.
Mathematics Subject Classification: 49Q20 / 34A60 / 49J24 / 93B03
Key words: Dynamic blocking problem / differential inclusion / constrained minimum time problem
© EDP Sciences, SMAI, 2009
Current usage metrics show cumulative count of Article Views (full-text article views including HTML views, PDF and ePub downloads, according to the available data) and Abstracts Views on Vision4Press platform.
Data correspond to usage on the plateform after 2015. The current usage metrics is available 48-96 hours after online publication and is updated daily on week days.
Initial download of the metrics may take a while.


